
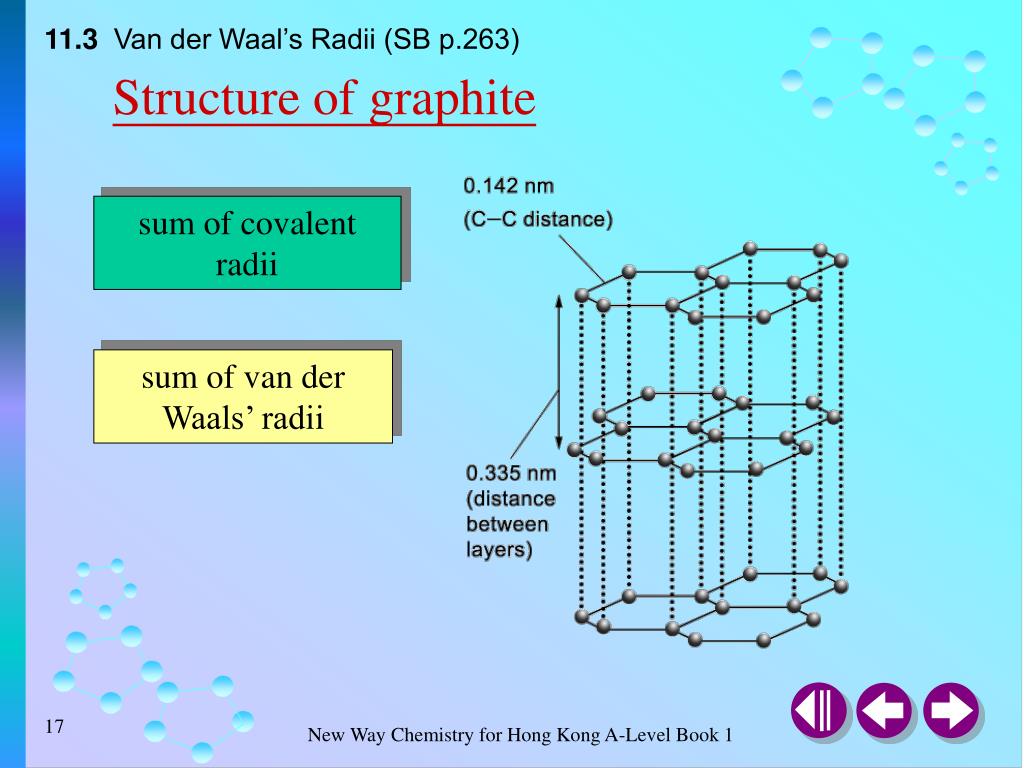

One of the basic concerns related to the interlayer vdW interaction is exfoliation. In general, the most acknowledged method to characterize accurate vdW interactions is still perturbation theory (PT) calculations. However, in complex systems, the vdW interaction can be affected by the local bonding environment and screening effect, thus making it harder to correctly characterize it with semiempirical methods. Moreover, there are still many other potential models extended from these two models. Similarly, if the exchange potential is modeled more accurately with the Born–Mayer term, then the well-known Buckingham potential is obtained.
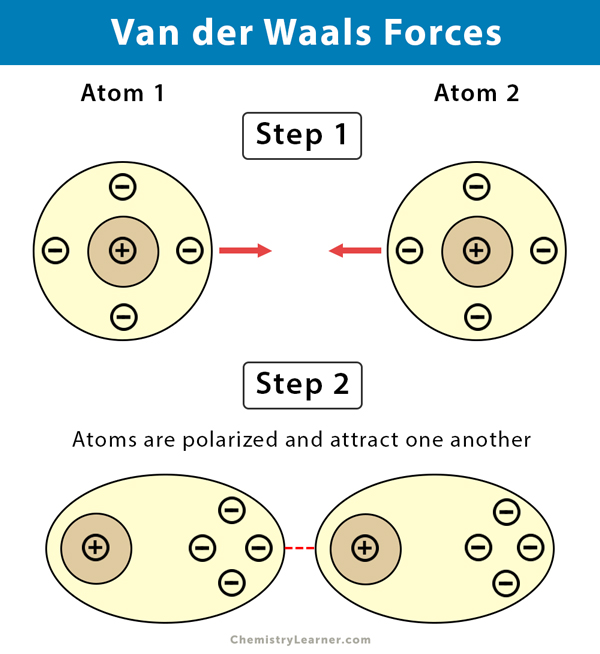
While taking only the first term of the series expansion as the vdW potential and modeling the exchange potential with an inverse power term, one obtains the famous Lennard–Jones (L-J) potential. From perturbation theory, the vdW potential is found to be a series expansion: − ∑ n ≥ 3 ∞ f 2 n R C 2 n 2 R − 2 n (where R is the distance) that should be terminated at different terms for different materials to achieve a certain accuracy. This characteristic of interlayer vdW interactions has enabled many fascinating properties and novel physical phenomena, such as flat-band and van-Hove singularities. The interlayer vdW interaction is far weaker than ionic bonding but strong enough to hold layers together and enable a certain degree of electronic coupling at the interface. The latter takes advantage of comparatively weak interlayer van der Waals (vdW) interactions. Interest in two-dimensional (2D) materials continues to grow due to their promising properties and the ability to arbitrarily integrate themselves.


 0 kommentar(er)
0 kommentar(er)
